
When faced with an unsolvable problem, you are said to face a “Gordian knot,” a rope that is tied so intricately or tangled so completely that it is impossible to untie. If you dive a little deeper into the story behind the legend, you learn that just because something is impossible doesn’t mean it doesn’t have a solution. This sounds like a lot of problems in aviation, so it is worthy of closer examination.
The Phrygians living in the southern Balkans sometime before the fourth century B.C. found themselves without a monarch, but an oracle decreed that the next man driving an ox cart into the city of Gordium would become their king. The new king’s son tied the ox cart to a post with the now famous knot. Years later another oracle said that any man able to unravel the elaborate knot would be destined to be the ruler of all Asia. Alexander the Great arrived and figured it out. The most common story is that he simply drew his sword and sliced the knot in half. Job done! Another version says that he pulled both ends of the rope from the cart and the post and pushed them into the knot, loosening it and allowing him to untie it. Once again, mission accomplished. (Spoiler alert: Alexander the Great went on to conquer Asia, fulfilling the prophecy.)

Legend or myth, the story of the Gordian knot is useful to us as aviators because it teaches us that there are two possible ways to deal with a seemingly impossible task. First, like Alexander’s “cut the knot into two” solution, we can redefine the problem to fit the solutions available to us. (We don’t need to untie the knot, only free the cart from the post.) Alternatively, like Alexander’s idea of freeing the rope from the post and the cart, we can discover new ways to solve old problems. (The problem isn’t the tether between the cart and the post, the problem is the knot itself.)
Redefine the Problem
We sometimes accept our problems as they are presented to us. Even as we invent new tools to handle other daily tasks, the old problems remain as we first found them. Take, for example, the problem of landing without vertical path information after flying an instrument approach, the classic “non-precision approach” landing from a minimum descent altitude (MDA). I still have my first Air Force instrument pilot’s manual, circa 1976. The only guidance given was this: “Arrive at the MDA with enough time and distance remaining to identify runway environment from MDA to touchdown at a rate normally used for a visual approach in your aircraft.” There was no mention of aiming for the normal touchdown zone or prohibition from descending early or attempting a landing when already halfway down the runway.
These types of “transition” approaches were the cost of doing business, after all. If the runway didn’t have an instrument landing system (ILS) or was served by a precision approach radar (PAR) system, you had no choice but to “dive and drive.” There have been countless examples of that turning into “dive and die,” but there was no easy solution to fix it short of installing an ILS or training pilots and controllers to use PARs. As we knew it, the problem was simply that non-precision approaches carried with them a higher risk of aircraft accidents.
But that wasn’t the problem at all. Redefined, the problem became: How do we transition from instrument flight conditions along an MDA to a stable descent in visual flight conditions to arrive above a runway touchdown zone properly configured to land?
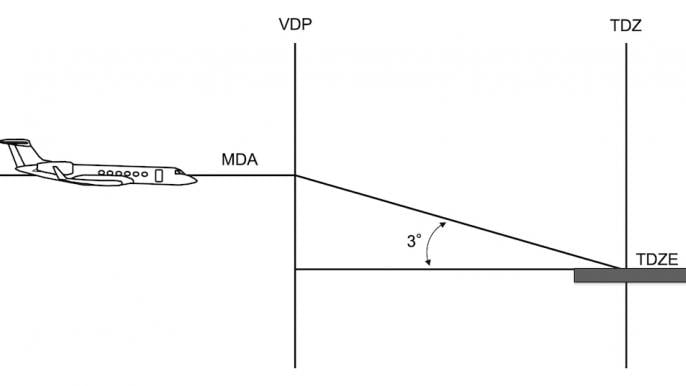
The first attempt to solve this problem required only that pilots be trained to figure out the point along the MDA where a normal descent to landing should occur. You simply subtract the touchdown zone elevation (TDZE) from the MDA and divide that by 300. The result is the number of nautical miles prior to the TDZ where you should start to descend from the MDA. Descending too early risks hitting approach lights (or something worse). Descending too late risks not having enough runway to stop. I thought for sure the use of visual descent points (VDPs) would end forever the dive and die approach. But they continued. (Never underestimate a pilot’s desire to descend as soon as the runway is in sight, or to salvage a late descent when there is still some runway left in view.)
Our redefinition of the problem needed a redefinition of its own. The real problem is this: How do you transition from instrument flight conditions to a stable descent in visual flight conditions to arrive above a runway touchdown zone properly configured to land? (The MDA isn’t a part of the new problem definition.)
The continuous descent final approach (CDFA) eliminates the drive part of the “dive and drive” and reduces the dive part to a similar descent rate found on an ILS. Aircraft with vertical navigation (VNAV) can synthetically concoct their own descent paths so that the airplane is aimed for the touchdown zone early on. There remains a problem, however, because the normal VNAV glidepath has tolerance that could under- or over-shoot the runway by a large margin. But we can fix that by looking at this age-old problem from an entirely different angle.
The next part of this article series will look at solving a problem from another angle.





